Table of Contents
Within the limits of the error of experiment, a definite volume of a solution or gas represents a certain weight of metal or other substance, hence the exact weight may be determined by experiment. The error of experiment may be reduced to insignificant dimensions by repeating the experiment, and taking the mean of three or four determinations. This will at the same time show the amount of variation. Thus, if 0.5 gram of iron were dissolved and found to require 50.3 cubic centimetres of the solution of permanganate of potash, and if on repeating, 50.4, 50.2, and 50.3 c.c. were required, the experimenter would be justified in saying that 50.3 c.c. of the permanganate solution represent 0.5 gram of iron, and that his results were good within 0.2 c.c. of the permanganate solution. So that if in an unknown solution of iron, 50.5 c.c. of the permanganate solution were used up, he could state with confidence that it contained a little more than 0.5 gram of iron. With a larger experience the confidence would increase, and with practice the experimental error will diminish.
But supposing that the unknown solution required, say, 100.5 instead of 50.5 c.c., he would not be justified in saying that, since 50.3 c.c. are equivalent to 0.5 gram, 100.6 c.c. are equivalent to twice that amount; and that, consequently, the unknown solution contained a little less than 1 gram of iron; or, at least, he could not say it except he (or some one else) had determined it by experiment. But if on dissolving 1 gram of iron, he found it to require 100.6 c.c. of the solution, and in another experiment with 0.8 gram of iron that 80.5 c.c. of the solution were required, he would be justified in stating that the volume of solution required is proportional to the quantity of metal present. There are a large number of volumetric assays of which this is true, but that it is true in any particular case can only be proved by experiment. Even where true it is well not to rest too much weight upon it, and in all cases the quantity of metal taken, to determine the strength of the solution used, should not differ widely from that present in the assay. There are certain terms which should be explained here. When the solution of a reagent is applied under such conditions that the volume added can be correctly determined, the operation is called “titrating,” the solution of the reagent used the “ standard solution,” and the process of determining the strength of the standard solution is “ standardising.” The “standard” is the quantity of metal equivalent to 100 c.c. of the standard solution.
Standard Solutions
In making these the salt is accurately weighed and transferred to a litre flask, or to the graduated cylinder, and dissolved. The method of dissolving it varies in special cases, and instructions for these will be found under the respective assays. Generally it is dissolved in a small quantity of liquid, and then diluted to the mark. For those substances that require the aid of heat, the solution is made in a pint flask, cooled, and transferred; after which the flask is well washed out. After dilution, the liquids in the measuring vessel must be thoroughly mixed by shaking. This is more easily and better done in the cylinder than in the litre flask. The solution is next transferred to a dry “Winchester” bottle and labelled. The label may be rendered permanent by waxing it.
Standard solutions should not be kept in a place exposed to direct sunlight. Oxidising and reducing solutions, such as those of permanganate of potash, ferrous sulphate, iodine, hyposulphite of soda, &c., gradually weaken in strength; the solutions of other salts are more stable; while those of potassium bichromate and baric chloride are almost permanent. Solutions of potassium permanganate may be kept for a month or so without much change. The solutions of hyposulphite of soda and of iodine should be examined weekly. Ferrous sulphate solutions, if acidulated with sulphuric acid, may be depended on for two or three weeks without fresh standardising. Before filling the burette, the “Winchester” bottle should be well shaken and a portion of about 50 or 100 c.c. poured into a dry beaker or test-glass. Besides the standard solutions, which are required for titrating an assay, permanent solutions of the metal or acid of equivalent strength are very useful. When the finishing point of a titration has been overstepped (i.e., the assay has been “overdone”), a measured volume, say 5 or 10 c.c., of a solution containing the same metal may be added. The titration can then be continued, but more cautiously, and the value in “c.c.” for the quantity added be deducted from the final reading.
Standardising
Suppose the object is to standardise a solution of permanganate similar to that referred to above. A convenient quantity of iron (say 0.5 gram) would be weighed out, dissolved in dilute sulphuric acid, and the solution titrated. Suppose 49.6 c.c. of the permanganate solution are required, then
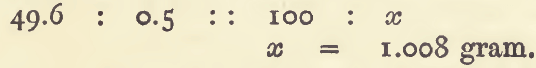
This result, 1.008 gram, is the “standard”. When a gas is measured, the standard may be calculated in the same way. For example : with 0.224 gram of zinc, 75.8 c.c. of gas were obtained. Then the quantity of zinc equivalent to 100 c.c. of the gas is got by the proportion.
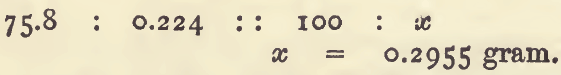
Using the term “ standard” in this sense, the following rules hold good:—
To find the weight of metal in a given substance :—Multiply the standard by the number of c.c. used and divide by 100. For example: a piece of zinc was dissolved and the gas evolved measured 73.9 c.c., Then by the rule, 0.2955 x 73.9 ÷ 100 should give the weight of the piece of zinc. This gives 0.2184 gram.
To find the percentage of metal in a given substance :—Multiply the standard by the number of c.c. used and divide by the weight of substance taken. For example: if 2 grams of a mineral were taken, and if on titrating with the permanganate solution (standard 1.008) 60.4 c.c. were required, then 1.008 x 60.4 ÷ 2 = 30.44. This is the percentage.
If the standard is exactly 1 gram, and 1 gram of ore is always taken, these calculations become very simple. The “c.c.” used give at once the percentage, or divided by 100 give the weight of metal.
If it is desired to have a solution with a standard exactly 1.0 gram, it is best first to make one rather stronger than this, and then to standardise carefully. Divide 1000 by the standard thus obtained and the result will be the number of c.c. which must be taken and be diluted with water to 1 litre. For example: suppose the standard is 1.008, then 1000 ÷ 1.008 gives 992, and if 992 c.c. be taken and diluted with water to 1000 c.c. a solution of the desired strength will be obtained. The standard of this should be confirmed. A simpler calculation for the same purpose is to multiply the standard by 1000 ; this will give the number of c.c. to which 1 litre of the solution should be diluted. In the above example a litre should be diluted to 1008 c.c.
It has been assumed in these rules that the titration has yielded proportional results; but these are not always obtained. There can be no doubt that in any actual re-action the proportion between any two re-agents is a fixed one, and that if we double one of these then exactly twice as much of the other will enter into the re-action ; but in the working it may very well be that no re-action at all will take place until after a certain excess of one or of both of the re-agents is present. In titrating lead with a chromate of potash solution, for example, it is possible that at the end of the titration a small quantity of the lead may remain unacted on; and it is certain that a small excess of the chromate is present in the solution. So, too, in precipitating a solution of silver with a standard solution of common salt, a point is reached at which a small quantity of each remains in solution ; a further addition either of silver or of salt will cause a precipitate, and a similar phenomenon has been observed in precipitating a hydrochloric acid solution of a sulphate with baric chloride. The excess of one or other of the re-agents may be large or small; or, in some cases, they may neutralise each other. Considerations like these emphasise the necessity for uniformity in the mode of working. Whether a process yields proportional results, or not, will be seen from a series of standardisings. Having obtained these, the results should be arranged as in the table, placing the quantities of metal used in the order of weight in the first column, the volumes measured in the second, and the standards calculated in the third. If the results are proportional, these standards will vary more or less, according to the delicacy of the process, but there will be no apparent order in the variation. The average of the standards should then be taken.
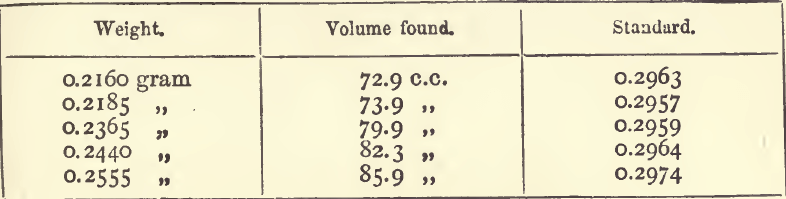
Any inclination that may be felt for obtaining an appearance of greater accuracy by ignoring the last result must be resisted. For, although it would make no practical difference whether the mean standard is taken as 0.2961 or 0.2963, it is well not to ignore the possibility that an error of 0.4 c.c, may arise. A result should only be ignored when the cause of its variation is known.
In this series the results are proportional, but the range of weights (0.216—0.2555 gram) is small. All processes yield fairly proportional results if the quantities vary within narrow limits.
As to results which are not proportional, it is best to take some imaginary examples, and then to apply the lesson to an actual one. A series of titrations of a copper solution by means of a solution of potassic cyanide gave the following results :—
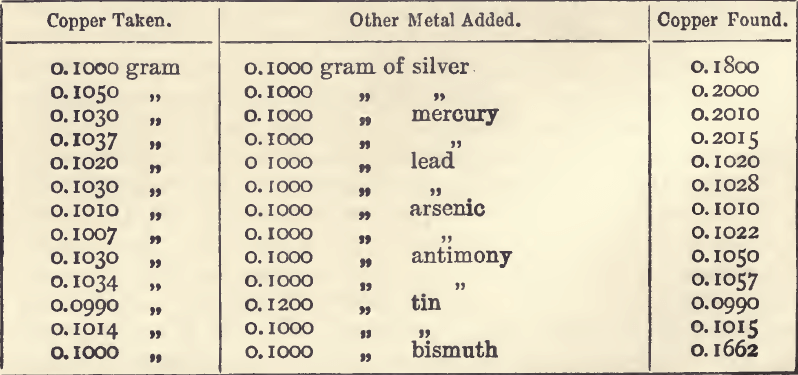
These are proportional, but by using a larger quantity of acid and ammonia in the work preliminary to titration, we might have had to use i c.c. of cyanide solution more in each case before the finishing point was reached. The results would then have been:
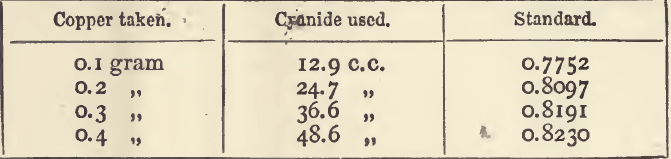
It will be noted that the value of the standard increases with the weight of metal used; and calculations from the mean standard will be incorrect.
By subtracting the lowest standardising from the highest, a third result is got free from any error common to the other two; thus:—

And the standard calculated from this corrected result is 0.8404. Further, if 0.3 gram requires 35.7 c.c., then 0.1 gram should require 11.9 c.c., or 1.0 c.c. less than that actually found.
We may therefore use the following rules for working processes which do not yield proportional results. Make a series of two or three titrations, using very different quantities of metal in each. Subtract the lowest of these from the highest, and calculate the standard with the remainder. Calculate the volume required by this standard in any case, and find the excess or deficit, as the case may be. If an excess, subtract it from the result of each titration ; if a deficit, add it; and use the standard in the usual way. The following table shows an actual example:—

It will be seen that the standard decreases as the quantity of chalk increases ; this points to a deficiency in the quantity of gas evolved.
Then
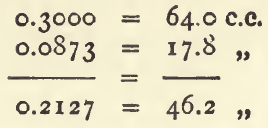
and 0.2127 x 100 ÷ 46.2 = 0.4604. Then, multiplying the weight of chalk taken by 100, and dividing by 0.4604, we get the calculated results of the following table:—
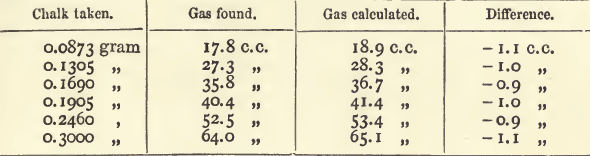
By adding 1 c.c. to the quantity of gas obtained, and taking 0.4604 as the standard, the calculated results will agree with those found with a variation of 0.1 c.c. When a large number of assays of the same kind are being made, this method of calculation is convenient ; when, however, only one or two determinations are in question, it is easier to make a couple of standardisings, taking quantities as nearly as possible the same as those present in the assays.
Sometimes it is necessary to draw up a table which will show, without calculation, the weight of substance equivalent to a given volume of gas or of solution. The substance used for standardising should be, whenever possible, a pure sample of the substance to be determined—that is, for copper assays pure copper should be used, for iron assays pure iron, and so on ; but when this cannot be got an impure substance may be used, provided it contains a known percentage of the metal, and that the impurities present are not such as will interfere with the accuracy of the assay. Including compounds with these, the standard may be calculated by multiplying the standard got in the usual way, by the percentage of metal in the compound or impure substance, and dividing by 100. If, for example, the standard 1.008 gram was obtained by using a sample of iron containing 99.7 per cent, of metal, the corrected standard would be 1.008 x 99.7 ÷ 100 = 1.005.
In volumetric analysis the change brought about must be one in which the end of the reaction is rendered prominent either by a change of colour or by the presence or absence of a precipitate. If the end of the reaction or finishing-point is not of itself visible, then it must be rendered visible by the use of a third reagent called an indicator.
For example, the action of sulphuric acid upon soda results in nothing which makes the action conspicuous ; if, however, litmus or phenolphthalein be added the change from blue to red in the first case, or from red to colourless in the second, renders the finishing-point evident. Some indicators cannot be added to the assay solution without spoiling the result; in which case portions of the assay solution must be withdrawn from time to time and tested. This withdrawal of portions of the assay solution, if rashly done, must result in loss; if, however, the solution is not concentrated, and if the portions are only withdrawn towards the end of the titration, the loss is very trifling, and will not show-up on the result. The usual plan adopted is to have a solution of the indicator placed in drops at fairly equal intervals distributed over a clean and dry white porcelain-plate : a drop or two of the solution to be tested is then brought in contact with one of these and the effect noted. Another plan is to have thin blotting-paper, moistened with a solution of the indicator and dried; a drop of the solution to be tested placed on this shows the characteristic change. When the assay solution contains a suspended solid which interferes with the test, a prepared paper covered with an ordinary filter-paper answers very well; a drop of the solution to be tested is placed on the filter-paper, and, sinking through, shows its effect on the paper below.
Except when otherwise stated, all titrations should be made at the ordinary temperature; cooling, if necessary, by holding the flask under the tap. When a titration is directed to be made in a boiling solution, it must be remembered that the standard solution is cold, and that every addition lowers the temperature of the assay.
On running the solution from the burette into the assay, do not let it run down the side of the flask. If a portion of the assay has to be withdrawn for testing, shake the flask to ensure mixing, and then take out a drop with the test-rod; the neglect of these precautions may give a finishing-point too early. This is generally indicated by a sudden finish, in which case on shaking the flask and again testing no reaction is got. Do not remove the drop on the point of the burette with the test-rod; let it remain where it is or drop it into the solution by carefully opening the clip.
Generally the methods of working are as follows :—
(1) When the finishing-point depends on a change of colour in the solution.—Increase the bulk of the assay up to from 100 to 150 c.c. with water. Boil or cool, as the case may be. Run in the standard solution from a burette speedily, until the re-agent appears to have a slower action, and shake or stir all the time.
Then run 1 c.c. or so at a time, still stirring, and finally add drops until the colour change is got.
(2) When an outside-indicator is used.—Pour the standard solution from a burette into the assay until 5 or 6 c.c. from the finishing-point; then run in 1 c.c. at a time (stirring and testing on the plate between each) until the indicator shows the change wanted, and deduct 0.5 c.c. for excess. When greater accuracy is sought for a duplicate assay is made. In this case the standard solution is run in close up to the end, and the operation is finished off with a few drops at a time.
(3) Where the finishing-point depends upon the absence of a precipitate and no outside-indicator is used.—As in the last case, run in the standard solution up to within a few c.c. of the end, then run in 1 c.c. at a time until a precipitate is no longer formed, but here 1.5 c.c. must be deducted for excess, since it is evident that the whole of the last “ c.c.” must have been, and a portion of the previous one may have been, in excess.
