Table of Contents
Conventional practice for treating mine drainage and other water sources contaminated with heavy metals is lime precipitation and settling of the hydrous oxides. This produces a voluminous toxic sludge that results in another type of disposal problem. In addition, settling of the precipitated hydrous oxides often does not produce sufficiently pure water to meet statutory limits for discharge, so the decant has to be polished by filtration through sand or other granular media. Metal hydroxides are difficult to filter because of their small size and their low resistance to the hydraulic shear forces encountered in conventional granular filters. Flocculation with organic polyelectrolytes is often necessary to achieve efficient filtration.
Magnesium oxide (MgO) is similar to lime (CaO) and can be used analogously to precipitate heavy metals. Although MgO is less soluble than lime at high pH, in acidic to slightly alkaline water it provides more neutralization capacity per unit weight than lime, owing to its lower molecular weight. When a stoichiometric excess of MgO is used to precipitate heavy metals from wastewater, the resulting sludge is up to 4 times more compact than that produced by liming. This was attributed to the unique positive electrokinetic charge of the MgO surface at neutral and slightly basic pH, in contrast to the negative surface charge of most metal hydroxides. The resulting electrostatic attractive force causes the fresh heavy metal precipitate to cement, or adhere to, the MgO surface and expel water molecules from the spaces between the precipitated particles, giving a denser solid.
Forms of Magnesia
MgO is often obtained from seawater or other brines that are rich in MgCl2. Lime is added to the brine to produce Mg(OH)2 precipitate and CaCl2 brine. The precipitate is dehydrated by calcining to produce MgO. Depending on the temperature and duration of the calcination, a multitude of MgO products can be obtained with different properties and reactivities. By calcining at lower temperatures (<700° C), much of the original porosity associated with the crystal structure of Mg(OH)2 is retained and a very active magnesia is produced. By calcining at higher temperatures (“dead burning”), the MgO is fused, the porosity is lost, and a tough, relatively inert, crystalline material is produced.
Periclase is a natural magnesium oxide mineral sometimes found in marble. It easily alters to brucite or hydrous magnesia, MgO·H2O, which is one of the many sources of dead-burned magnesite. Granular, dead-burned MgO has been demonstrated to be an efficient deep-bed filter medium for removing particulates flocculated with aluminum salts. In previous research, pure MgO powder was used as a precipitant to remove heavy metals. One objective of this research was to find whether granular periclase can also precipitate heavy metals, thereby achieving both precipitation and filtration steps in the same bed. It was found that the granular filter material has sufficient activity to raise the pH of unbuffered water several units. At typical flow rates in deep-bed filters the effluent can have a pH of 10 or greater when the influent pH is about 7. This increase in pH is enough to cause heavy metals to precipitate as oxides or hydroxide as they pass through the filter.
These precipitates were observed to be quite strongly attached to the MgO granules. One objective of this study was to determine if the presence of dissolved heavy metals in the influent would impair the ability of the MgO to filter suspended solids. Early results indicated that significant metals removal occurred in the filter, so the second objective was to measure the metals removal capacity of the MgO and to test the feasibility of removing both suspended solids and dissolved metals in the same bed. The third objective was to determine the feasibility of recovering these metals by eluting them from the loaded MgO bed with either chelating agents or acids.
Electrical Phenomena at Interfaces
The surface charge on metal oxides or hydroxides in water is pH dependent and is described by Lippmann’s equation:
![]()
where σ is the surface charge density, i.e., charge per unit surface area, γ is the interfacial tension, and E is the interfacial potential. The derivation of equation 1 is given in the appendix.
The electrocapillary curves in figure 1 show the variation in surface charge with respect to pH (or interfacial potential) for a silicate mineral such as amphibole asbestos and for MgO. The maximum of each curve corresponds to a surface charge density of zero, and the pH resulting in zero net surface charge is called the zero point of charge (ZPC). The surface charge of a metal oxide is therefore dependent on the pH and moves from positive to negative with increasing pH, becoming zero at some intermediate pH. A method was developed

to remove asbestos fibers from water, at pH intermediate to the ZPC of MgO and asbestos, based on the electrokinetic attractive forces between the unlike surface charge on asbestos and MgO.
The ZPC values vary from oxide to oxide and correspond to the pH of minimum solubility of each metal oxide. In general, oxides with cations in higher oxidation states are more acidic and will have a lower ZPC. The ZPC is mainly dependent on the ratio of charge (Z) to radius (R) of the cation in the pure solid. In a simple electrostatic model the ZPC decreases with increasing ionic potential, Z/R, and corrections for crystal field effects (coordination number) are made to refine the model. The ZPC’s of various metal oxides were compiled by Parks and are shown in table 1. Depending on the measurement method employed and the exact sample preparation, the reported values of ZPC for some of the metal oxides vary over a wide range.

The Stern model (fig. 2) of the electrical double layer presents a fairly simple and useful picture of the electric potential around a solid in water. It predicts electrical potential based on the ionic double-layer theory. The electrical potential difference, E0 , that develops between the solid surface and the bulk solution is the balance between electrostatic attraction of the solution counterions to the solid surface and their tendency to diffuse away from the surface. In the absence of specific adsorption, the equilibrium concentration gradient of the potential-determining ions creates the electrical potential shown in figure 2. The distance over which there exists a concentration gradient is very small. The double-layer thickness, 1/k, is a function of ionic strength and temperature. At room temperature, for example, in a 10 -5 M NaCl solution, 1/k is about 0.1 µm, or 1,000 A. Since OH- and H+ are potential-determining ions, the pH at the solid surface can be quite different from that measured in the bulk solution. For an electrical potential of less than 25 mV, this difference can be approximated (with some qualifications) by

Colloid stability theory considers the net effect of the London-van der Waals force of attraction and the interaction of ionic double layers, which can produce attractive or repulsive forces. The total potential energy of the particles is a function of their separation distance (fig. 3). For strong electrostatic attractions, a primary minimum in the potential energy curve exists very close to the solid surface (the global minimum), A secondary minimum may exist at about four times the equivalent thickness of the double layer, 1/k. The exact positions of these two minima depend on the electrokinetic properties of the embryonic metal precipitates (and hence on the nature of the heavy metal) as well as on the available MgO surface.


Depending upon whether there are (a) strongly favorable (unlike charges), (b) weakly favorable, or (c) unfavorable (like charges) ionic double-layer interactions, a nascent precipitate will be respectively (a) cemented, (b) weakly adherent, or (c) dispersed away from the solid surface. Under the right conditions, favorable surface interactions are important for capturing suspended solids in granular filters.
Dual Role of MgO as a Filter and Precipitant
Flow in conventional water filters is usually laminar (or transitional), and the granules of filter medium are surrounded by a boundary layer of relatively stagnant fluid. In a water filter the chemical conditions near the surface of the MgO granules may vary considerably from that of the bulk solution. Not only does the pH of the bulk solution increase as a result of passing through the filter, but ions and particles that cross streamlines to be captured by the MgO encounter an increase in the Mg2+ and OH- concentrations. Dissolved heavy metals will precipitate in this region before the pH in the bulk solution would be raised sufficiently to cause insolubility and precipitation. The charge on the newly precipitated metal hydroxide particles will depend on the local pH level and will tend to become more negative as the particles approach the MgO surface, owing to the increasing pH. The more soluble or mobile metals will form hydroxides near the MgO surface where the ionic double-layer interactions are the strongest and will be more strongly bound than ordinary filter deposits, because the MgO surface is positively charged (ZPC of MgO is 12.4, table 1). Strong bonding should be related to the solubility product and the ZPC of the individual metal hydroxides.
Removal of heavy metals in the MgO filter can be thought of as an ion-exchange process in which 1 mol Mg is exchanged for each mol of M2+. Any CaO impurity in the MgO material will also contribute. Metals either pass through the filter or are bound as metal oxides or hydroxides, Depending upon how tightly they are bound, the metals retained in the filter either will be removed by conventional backwashing or else will accumulate on the filter over successive cycles of filtration and backwashing. Weakly bound metal hydroxides will be removed in normal backwashing, while strongly cemented hydroxides will need chemical stripping by dilute mineral acid or chelating agents such as ethylenediamine-tetraacetic acid (EDTA) or other selective leachants. Preliminary experiments showed that a 1-pct EDTA solution recovered metals as efficiently as 1-pct HCl; however, the dissolution of MgO was much less with the EDTA. Because of this, EDTA was chosen as the stripping reagent in this work. Acidifying the EDTA solution containing the stripped metal precipitated its hydrogen form, which was filtered out and then reacted with NaOH to regenerate its soluble sodium form. The objectives of this re-search are therefore not only to use granular MgO for the combined precipitation and filtration of heavy metals, but also to investigate the recovery of the precipitated metal values from simulated mining and mineral processing waste streams.
Experimental Procedure
Filter beds were composed of granular periclase (MgO) from Basic Chemical. This is a dead-burned product obtained from high-temperature calcining of Mg(OH)2 derived from a seawater brine. This filter material assayed, in percent, 95.7 MgO, 2.8 CaO, 0.8 SiO2, 0.3 Al2O3, and 0.3 Fe2O3. Two experimental filter systems were used. The first system was composed of three 2.5- by 25-cm acrylic columns in series, each filled to a depth of 15.2 cm with 0.5-mm MgO. The second filter was a 5.0-cm-ID column of acrylic plastic about 1 m in height with a 46-cm bed of 0.5-mm MgO. Support for the medium was 50-mesh stainless steel screen. Filtration velocity through both beds was 0.34 cm/s.
Stock solutions of each of the heavy metals employed were prepared with distilled water and contained 5.0 g/L of each heavy metal. Reagent-grade hydrated nitrate or sulfate solids of the various heavy metals were used to make these solutions. The appropriate volume of stock solution was diluted to 100 L in a large plastic reservoir with tap water filtered through PALL MCY1001NAE cartridge filters. Manganese was available as a reagent-grade 50-pct-Mn(NO3)2 solution; the appropriate volume of this solution was pipetted directly into the reservoir. Influent was pumped by one channel of a dual-channel Masterflex pump. Dilute HCl or HNO3 solution was added via the second channel as needed to maintain the influent at a pH of 7,0 as it entered the filter. The dilution due to the second channel was about 4 pct and was approximately compensated when the stock solutions were added to the reservoir. Influent concentrations of the metals were below the solubility limit at pH 7.0 calculated from solubility products.
Filtrate turbidity was measured at 15-min intervals with a Hach 2100A turbidimeter. Influent turbidities were measured at the beginning and end of the tests, which lasted 6 h. Filtrate pH measurements were made concurrently with turbidity measurements. pH was measured with an Orion 901 Ionanalyzer or an Orion 601 pH meter using a 91-02 double- junction, research-grade glass electrode. Filtrate samples were taken at 30-min intervals and analyzed chemically by atomic absorption spectroscopy (MS) together with the influent samples. Aliquots were preserved with 0.5 mL HNO3 per 25-mL sample to prevent post precipitation of the heavy metals. The lower level detection limit for the direct atomic absorption analysis was 0.1 mg/L (0.1 ppm).
Two methods of backwashing were used. For the three-column unit the contents of each column were strongly agitated by roller tumbling for 10 min in a 500-mL jar containing approximately 200 mL of (1) distilled water or (2) 1-pct EDTA solution. Each column went through four to six cycles of cleaning, and samples of each backwash were acidified and analyzed by AAS. The larger filter column was backwashed by a method similar to conventional water treatment practice: 30 min of air-assisted backwash followed by 30 min of high-rate fluidization at 50-pct bed expansion. Backwashing with a mixture of compressed air and water agitates the bed vigorously and is often used in practice. Residual metal values were stripped with an upward flow of 1- pct EDTA solution at a rate of 500 mL/min (0.43 cm/s), which was less than the minimum fluidization velocity. Backwash samples were withdrawn at 20-min intervals for chemical analysis.
Results and Discussion
Heavy-Metal Retention of MgO Filter Beds
The three-column filtration unit was packed with 1.09-mm granular periclase as the filter medium. An influent solution containing 0.8 mg/L Cu, 3.2 mg/L Cd, and 3.6 mg/L Zn at pH 6.9 gave an effluent containing <0.1 mg/L of each of the three metals for the first 15 min. During the 5-h duration of this experiment, Cu and Zn in the effluent remained <0.1 mg/L, but the Cd concentration rose gradually from 1,0 mg/L after 100 min to 1.8 mg/L at the end of the test. The filtrate pH dropped from 9.29 at the beginning of the test to 8.94 at the end. Pressure drop across each of the three columns rose only 0.18, 0.07, and 0.07 m H2O, respectively, during the test. It appears that the pH was not kept high enough throughout the MgO beds to precipitate all of the Cd, which has the highest solubility product of the three metals (table 1).
A duplicate test employing 0,50-mm periclase grains instead of the 1.09-mm grains enabled the bed pH to be kept at a higher level for a longer time. The result was a filtrate containing less than 0.10 mg/L of the three metals, with the Cd concentration remaining at that level for the first 90 min, instead of the 15 min for the coarser MgO. Also, after 5 h the filtrate contained only 0.39 mg/L Cd, compared with 1.8 mg/L Cd with the 1,09-mm MgO grains.
To maintain enough precipitation ability for MgO without sacrificing its filtration capacity, all subsequent tests were performed with 0.50-mm MgO grains.
A synthetic waste stream containing approximately 1 mg/L Cu, 4 mg/L Cd, and 4 mg/L Zn was passed through the three-column unit. The influent pH was kept between 6.9 and 7.2. Filtrate pH decreased steadily with successive runs when only water backwashing was used. Data for this series of tests are shown in figure 4A. Very little Cd was retained on the column after one loading cycle, the Zn broke through after two loading cycles, and Cu was retained until the fifth loading cycle. About 150 bed volumes were passed through the filter in each loading cycle except the third, which was cut short because the columns were blinded with air bubbles. The data indicate that the filter bed was saturated with metals after five cycles of loading and that Cd, the most mobile
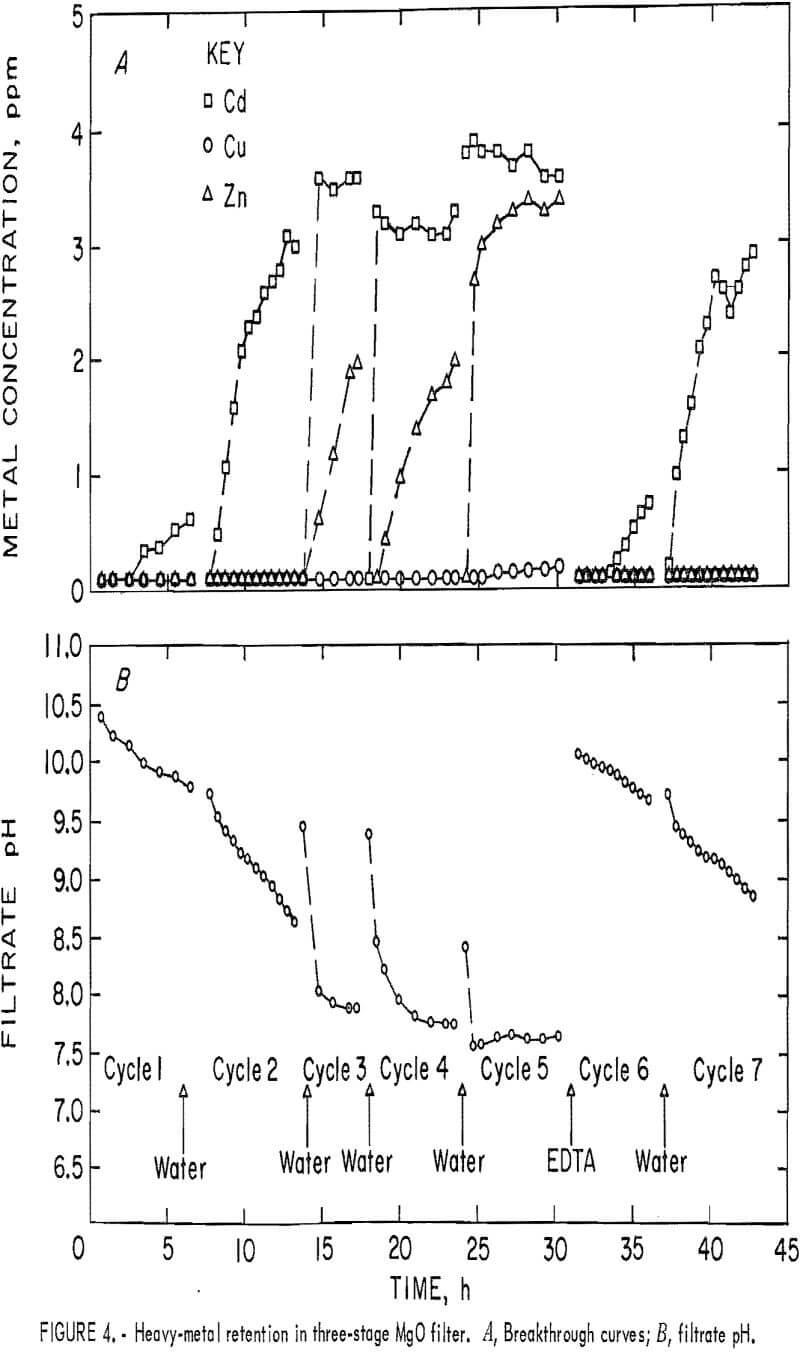
of the three heavy metal cations, broke through first, followed by the second most mobile cation, Zn, as would be expected. EDTA backwashing restored the performance of the filter to its original level or better, as indicated by the higher filtrate pH and better metals retention in the sixth loading cycle (fig. 4B).
Two synthetic waste suspensions, one containing only 25 mg/L suspended kaolin and the other containing 4.5 mg/L Zn, 3.7 mg/L Cd, 5.0 mg/L Ni, and 6.3 mg/L Mn in addition to the 25 mg/L suspended kaolin, were alternately flowed through a 5-cm-diam Plexiglas column containing 45.7 cm of 0.50-mm periclase grains at a rate of 400 mL/min for 6-h intervals. Starting with the plain kaolin suspension, a total of 30 h of filtration (three 6-h runs with plain kaolin solution and two 6-h runs with kaolin and heavy metals) was accomplished. After each 6-h interval the column was water-backwashed with air scouring. Filtration of kaolin solution resulted in pH drops of 0.25, 0.15, and 0.24 unit, and increases in bed pressure of 0.20, 0.14, and 0.07 m H2O. Influent turbidities in these tests were 16 NTU, while final turbidities were 0.77, 0.84, and 0.34 NTU.
Filtration of kaolin with heavy metals resulted in pH drops of 1.62 and 1.64 pH units and increases of bed pressure of 5.17 and 4.65 m H2O. Influent turbidities in these tests were 17 NTU, while final turbidities were 0.08 and 0.26 NTU. In both of these tests the Zn was almost completely removed (<0.1 mg/L) throughout the 6-h tests. Filtrate Cd concentration was initially less than 0.1 mg/L in both tests, but rose to about 1.0 mg/L at the end of one test and to about 4 mg/L by the end of the other test. Mn concentrations remained above 1 mg/L throughout the tests. The large drops in filtrate pH indicate that the pH was not always high enough to completely precipitate metals with higher solubility product (Ksp) values, i.e., Mn, Ni, and Cd. The presence of suspended solids apparently does not affect heavy metals removal significantly, nor does the presence of heavy metals seriously impair the filtration of suspended solids. In fact, results suggest that the dissolved metals may aid the removal of suspended solids. If the metals are periodically stripped from the bed so that the filtrate pH remains sufficiently high, metals removal and filtration can be done simultaneously in the same bed. The precipitation of heavy metals resulted in lower filtrate turbidity throughout the 6-h tests but also increased the pressure drop across the bed. Influent containing highly soluble metals such as Cd, Mn, and Ni requires maintaining higher filtrate pH to achieve adequate removal. This dictates the use of either deeper beds or finer sized MgO to supply extra surface area for metals precipitation.
Stripping of Heavy Metals from MgO Filters
A synthetic waste stream solution was passed through the three-column unit containing 0.5-mm MgO at a rate of 100 mL/min for 6 h. This solution contained 2.5 mg/L Al, 2.2 mg/L Cd, 2.1 mg/L Co, 1.0 mg/L Cr, 1.8 mg/L Cu, 1.9 mg/L Fe, 1.4 mg/L Mn, 1.8 mg/L Ni, 1.4 mg/L Pb, and 1.8 mg/L Zn and had an influent pH of about 6.9. All the metal values were almost totally removed (<0.1 mg/L) throughout the 6-h test. The filtrate pH dropped from 10.51 at the beginning of the test to 9.68 at the end. Each column was backwashed once with water and five times with 1-pct EDTA solution. The results of these tests are in table 2. Recoveries of the 10 elements from the MgO column ranged from 93.6 to 97.7 pct (water plus EDTA backwashing). For Al, Co, Cd, Cu, Mn, Ni, Pb, and Zn, the majority of each metal was recovered in the EDTA backwash. For Fe and Cr, the majority of each metal was recovered in the water backwash. Except for Mn, Cd, Al, and Co, a substantial amount (53 to 88 pct) of the metal was collected in the first column. For Co and Al, only about half of the metal value is collected in the first column. Cd was equally collected on the first and second columns, while more Mn was collected on the second column than on either the first or third column.

The quantity of each metal retained on the columns was calculated by numerically integrating the under the concentration versus time curves for the filtration tests, then multiplying by the volumetric flow rate (which was maintained at 100 mL/min) to get the total amount of each metal passing through the filter but not being captured. This was subtracted from the product of the influent concentration times the flow rate times the total run length to obtain the net amount retained oil the columns. Many of the reported concentration values for the effluent were below the detection limit of the chemical analysis; a value of 0.1 mg/L was used for these points in the integration process. The quantity of each metal stripped from the column was calculated by summing the products of back-wash volumes and reported concentrations from each column.
For each metal, the fraction recovered during the EDTA backwash relative to the total recovered by water plus EDTA back-wash is plotted versus ZPC values in figure 5. The brackets span the range of ZPC values selected by Parks from the literature and given in table 1. Aside from AI(III), the percentage recovery of metals increases with increasing ZPC, as would be expected since the higher the ZPC, the higher the pH of minimum solubility of the metal and the more mobile the metal ion. Apparently, precipitation of the more mobile ions

occurs closer to the MgO surface, probably at the distance corresponding to the global potential energy minimum of figure 3. Here the pH is higher and bonding of the metal hydroxide or oxide to the MgO is highly probable so that chemical stripping of the metal oxide or hydroxide is required. A straight line is drawn through as many of the bracketed values of ZPC as possible; at this time no theoretical importance has been attributed to the slope, intercept, or linearity of the plot. Aside from Al(III), only Cd(II) fails to contact the line. Only a single value was reported for Mn(II), so no bracketing values are available. The recovery of Cd(II) appears to be lower than it should be in these tests as results described later indicate.
A synthetic waste solution containing about 1 mg/L Cu, 4 mg/L Cd, 6 mg/L Mn, and 4 mg/L Zn was passed through the single 5-cm-diam MgO filter column at a rate of 400 mL/min for 6 h. The column was not allowed to saturate, as was done with the three-column unit. After each cycle of loading the filter was back-washed with air and water, then stripped with 1—pct EDTA solution. The metals eluted off the column quickly, as shown in figure 6. The area under each curve was determined by numerical integration and multiplied by the volumetric flow rate to obtain the total recovery of each metal. Two duplicate tests were run, and results are given in table 3. Most of the Cu was removed by water and

air backwashing, which accounts for the low Cu recovery by EDTA stripping. Recovery efficiency is much better for the Zn, Cd, and Mn by this method than by tumbling. The coating of metal hydroxides is dissolved, and the heavy metals elute off together in a concentrated solution (as evidenced by the peak in the concentration versus time plot) . This quick elution of the heavy metals means that the EDTA solution has limited con-tact time with the MgO; therefore MgO dissolution is kept at a minimum.
The total amount of Mg and Ca leached out of the column by the EDTA solution was determined by the same methods used for the heavy metals. Totals of 1.34 and 1.62 g Mg and 0.54 and 0.25 g Ca were dissolved by the EDTA in the two tests.

About 1.67 and 1.80 total grams of heavy metals were stripped from the column. On a molar basis, this amounts to 72 mmol (Ca + Mg) to 26 mmol total heavy metals stripped.
The efficiency of the process 160 bed volumes of some of the most mobile metal cations were successfully treated in each cycle before breakthrough. During filtration about 2 mmol (Ca + Mg) (about 50 pct each on a molar basis) is exchanged per total millimoles of heavy metals. The metals can be recovered in less than 8 L EDTA brine, and the metals are concentrated as much as twenty fold, with the exception of Cu, which is removed by normal backwashing. It is obvious from figure 6 that the bulk of the metals tripped within 6 to 8 min, and thus a volume equal to about half that actually used would be sufficient for metals recovery.
Metals recovery is apparently a strong function of ZPC, as shown in figure 5 for Cd, Co, Cr, Cu, Fe, Mn, Ni, Pb, and Zn, and in figure 7 for Cd, Cu, Mn, and Zn. The EDTA backwash data plotted in figure 7 are the average of both tests reported in table 3. The vertical brackets span the range of experimental values. The data fit very well to a straight line, suggesting a strong correlation between adherence to the MgO surface and the ZPC of the precipitate.
Several factors probably influence the ZPC and bonding of the heavy metals to the MgO. In the test with a stock solution of 10 heavy metals, the initial turbidity was higher than that for the 4 metals, indicating that more precipitate was formed in the 10-metal filter influent then in 4-metals influent. These precipitates, once formed, would tend to be captured by the mechanisms operating in conventional filtration. Normal backwashing with water is sufficient to remove these hydroxide precipitates so that the quantity of metals requiring removal by EDTA is lowered. Secondly, the ZPC is affected by defect structures and impurities (water, anions, and cations) occluded in the precipitate. Various metals could be coprecipitating,

which would explain why Al(III) recovery is unexpectedly high in the test results of figure 5. Interactions between 4 metals are diminished with respect to those possible for 10 metals, so the differences in stripping behavior are more pronounced. Thirdly, it should be pointed out that the precipitates are nascent precipitates; i.e., they are formed and captured within minutes or even seconds. There is most likely not enough time to form equilibrium structures, a process that may require days. The precipitates are likely to be disordered and to contain H2O at the expense of OH- or O=, as well as other impurities. The more ordered, high-purity structures with OH- and O= replacing H2O tend to have lower ZPC, so the nascent precipitates may be bonded more strongly than expected, based on ZPC values determined for aged precipitates.
Summary and Conclusion
In laboratory tests with synthetic solutions the metal concentrations of the solutions have been reduced to less than 0.1 mg/L by passage through a bed of granular dead-burned MgO. Recoveries of heavy metals from the MgO bed in excess of 95 pct were obtained by combined water backwashing and stripping with EDTA solutions. The granular MgO precipitation-filter process appears to have merit for removing and recovering heavy metals from waste streams that have been at least partly neutralized by liming. The metal concentration of the waste stream to be treated cannot be too high, nor can the pH of the waste stream be too low; otherwise the reactivity of the dead-burned MgO will not be sufficient to treat the wastewater, or excessive dissolution of the MgO will occur. In acid mine drainage it is fairly standard practice to neutralize to pH 7.0 and aerate to remove the iron. This is not sufficient to remove the more soluble heavy metals. With the granular MgO filter, heavy metal removal could be accomplished without further addition of lime or flocculant. The MgO filter is easily cleaned, and the metals recovery is relatively unaffected by the presence of suspended solids, such as occurs with ion-exchange columns. Fine tuning of the procedure and reducing the dead volume in the column could improve the heavy metal removal and recovery. By employing more columns of MgO and varying the reactivity of the MgO, the particle size of the MgO granules, and the depth of the MgO bed, it may be possible to improve the removal of the most mobile heavy metal ions and at the same time segregate the metals on different columns for easier recovery. It may also be possible to selectively strip and separate the metals from one column by using a series of metal-specific eluants. Metal values removed from the column by normal backwashing can be collected in a small volume of solution and then acidified to give a concentrated solution. Taking into account the OH- gradient around the MgO granules and the ZPC of the metals explains at least in part the need for chemical stripping of the more mobile metal cations from the beds of MgO.
