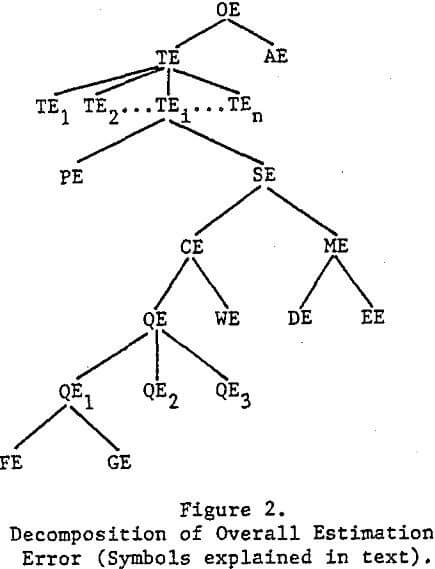
Diagrams Gy’s decomposition of the overall estimation error (OE). OE is the difference between the grade of the lot of particulate material and the grade of the sample. OE consists of two components: Total sampling error (TE) and analysis error (AE). The theory concentrates on TE. TE consists of preparation error (PE) and sampling error (SE). PE is handled qualitatively – essentially how to avoid preparation errors. The quantitative analysis is focused on estimating the mean and variance of SE both theoretically and practically.
Gy divides all particulate sampling problems into increment processes and spliting processes. Both are analyzed quantitatively. (Because splitting shares much in common with increment processes, the following discussion will concentrate on increment sampling.) The increment sampling process consists of four steps: 1) point selection (selecting the times for increment extraction), 2) increment delimitation (determining the theoretical boundaries of each increment while disregarding the particle structure), 3) increment extraction (actual realization of the increment which must take particle structure into account), and 4) increment reunion (creating the final sample by combining the individual increments).
When extraction is correct (a term precisely defined in the theory), a variographic experiment can be carried out to estimate the components of QE and WE and hence CE. Determination of the estimation variance of CE is nothing other than the determination of the sampling precision and takes the one-dimensional time correlation into account when sampling one-dimensional moving streams. When this approach is used to analyze various sampling strategies, random sampling is shown to be significantly inferior compared to systematic sampling and stratified random sampling..

