Increasing capacity of bulk material handling systems creates difficult problems for accurate in-line material weight measurements. At the same time increasing system complexity and growing user demands call for more measurements.

Precision in-line weight measurements for bulk materials are usually accomplished by application of conveyor belt scales.

Belt scale accuracies listed by scale vendors are applicable for ideal situations with suitable conveyor design, belt scale location and calibration means.

This paper attempts to provide designers and users with simple means to evaluate belt scale accuracy for practical scale installations.

Based on known mathematical models simplified formulas for measurement errors are derived and discussed. These formulas allow for simple determination of achievable accuracy for alternative scale installations on the conveyor, conveyor designs, etc.

The purpose of this paper is to provide simplified formulas and procedures for the quick determination of the belt scale accuracy in a practical application.

The belt scale accuracy problems are discussed in-depth in H. Coljin’s book titled “Weighing and Proportioning of Bulk Solids”.

Many details, practical rules and considerations for the belt scale installation, excluded from the scope of this paper, are efficiently covered in that book as well as in many belt scale vendor installation manuals.

The paper categorizes measurement error components and centers on a major problem area, i.e. errors due to the belt effects.

A comprehensive abbreviations table is included in the body of this paper being a key to understanding of the following mathematical formulas and procedures.

Several simplifying assumptions in this paper are introduced to clarify the problems.

The basic assumptions are as follows:

– conveyor belt tension at the tail pulley remains the same for all operating loads and head pulley tensions; practically this means that conveyor is equipped with a gravity take-up. Small changes in the tail pulley tensions are not taken into, consideration.
– material rests on the belt at the belt scale location, i.e. scale is far enough from the loading point.

Conveyor Belt Scale Accuracy

Belt scale accuracy depends on the following:

– scale hardware including the scale, scale integrating system and calibration system.
– conveyor design, scale location and installation on the conveyor.

The accuracy and stability of the belt scale hardware depends on the scale system design and quality. The scale hardware can be considered to be a source of a certain small fixed error of weight measurement.

The conveyor design, belt scale location and installation on the conveyor determine the effectiveness with which the scale can interpret material loading on the belt. This effectiveness is diminished by the so-called “belt effects” related to variations in belt tensions and stiffness combined with the degree of misalignment between the scale idlers and adjacent stationary idlers.

Belt effects contribute to small fixed errors for calibration conditions and large variable errors due mainly to tension changes after the scale has been calibrated.

This paper focuses on measurement errors due to the belt effects. These errors determine the practical belt scale performance.

Conveyor Belt Effects

The material on a belt transmits its weight through the belt system to the scale. Belt stiffness, troughing and tension create a resistance to longitudal bending in the belt. These factors, combined with misalignment between scale idlers and adjacent conveyor idlers, allow for partial supporting of a load. This physical supporting of a load is a major source of the belt scale in accuracy. Mathematical models have been developed for calculation of belt scale errors due to the belt effects. Formulas derived from one such model, developed by H. Coljin, are used in this paper.

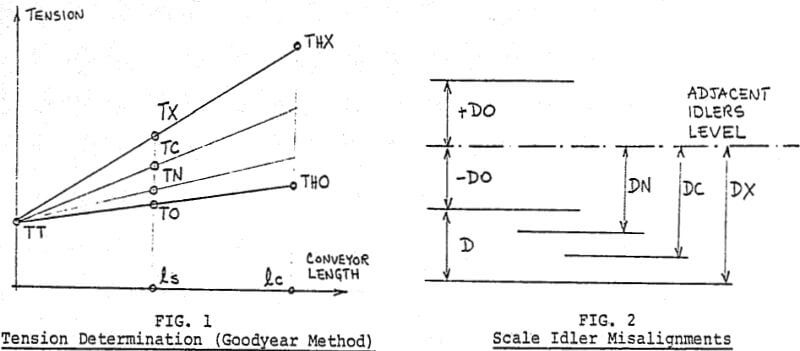
Practical Procedures

All the above-calculations can be easily performed on a programmable calculator with program stored on a magnetic card.

Calculation procedures can be simplified to the printed input comprising belt, scale and conveyor data, p% and d% values and calculated E%N and E%X printout.

It is recommended to assign p% values to the maximum load tension TX only. For p% and d% equal to zero errors for calibration conditions will be obtained. Then for assumed p% after-calibration tension changes and/or d% initial idler misalignment changes, practical weight measurement errors can be evaluated.

Conclusion

By using procedures described in this paper, designers and users of belt scale measurement systems can quickly evaluate achievable accuracy for alternative scale installations.