Table of Contents
It has already been shown by Maclaurin, that the dissolving power of a cyanide solution saturated with oxygen increases with its strength until a strength of 5 or 10 per cent, is reached, and diminishes again as the strength in cyanide increases beyond that point. But, so far as I am aware, no one has proposed the question: “At what point of dilution does the cyanide solution cease to act on the gold ?”
According to the Nernst theory, gold should cease to dissolve in cyanide solutions, provided no force acts except its own solution-pressure, at the point at which its electromotive force is zero, for then its solution-pressure will be just balanced by the osmotic pressure of the ions already in solution. At this point (provided no other force acts) the solution of the gold should cease.
It seemed interesting to ascertain if there were such a point. In order to do so, it was necessary to expose the gold to the cyanide solution, in the presence of air, under circumstances most favorable for rapid solution. Hence I devised a rotating apparatus, consisting of three pairs of rollers, driven by a small Pelton water-motor, on which a couple of 2½-liter bottles, such as are used for holding nitric acid, could be laid and rotated about their long axes. The number of revolutions of the middle axis being recorded, the distance traveled was known. This precaution was taken to be able to allow for the irregularities of the motor.
Standard strips of fine gold were prepared by repeated precipitation with sulphurous acid from a diluted chloride solution. These were rolled out thin and cut to a standard size of 2 in. by ¼ in. They weighed from 250 to 330 mg., according to their thickness. The strips were boiled in sulphuric and muriatic acids, washed, and ignited before use. The first set of experiments was undertaken with 2 liters of solution and ½ liter of air, the bottles being stoppered. The weighed gold strips were then added; the bottles were rotated for 24 hours; and the strips were then washed and dried and weighed again. The number of rotations made in 24 hours ranged from 4000 to 24,000, and as the interior diameter of the bottles was 4½ in., the distance traveled in this time by the gold strip was from one to six miles. It was found impossible to get a uniform rotation-rate, owing to constant changes in the water supply. But so long as the solution was kept gently agitated these variations did not seem to have any appreciable effect on the result.
Table XIII. shows the results of these experiments. The first pair were undertaken with distilled water, to see if there was any loss due to erosion. The apparent loss of 0.01 mg. was almost at the limit of accuracy of the balance, but seemed to show the possibility of a slight loss due to that cause. It will be observed that up to M/2000 or 0.00325 per cent, the gold-loss is merely nominal, never more than 0.29 mg., often zero ; and the results vary in the most irregular manner. No. 18, with M/10,000 or 0.00065 per cent., gave a loss of zero, and No. 20, with M/4,000 or 0.0016 per cent., only 0.08 mg. It is believed that these small losses below M/4,000 were chiefly mechanical. It was noted that while most of the bottles used were perfectly smooth inside, some seemed to have small sharp grains of sand, or slivers of glass, projecting above the smooth inner surface. In many cases it was impossible to detect these without breaking the bottles. The loss in No. 12, which was not rotated, cannot be set down to this cause. The explanation in this case, and perhaps in some others, may have been an imperfect mixing of the solution. The solutions were made up by adding the proper volume of strong solution to the proper amount of distilled water. In case the mixture of the solutions was not thoroughly made before the gold strip was added, the gold
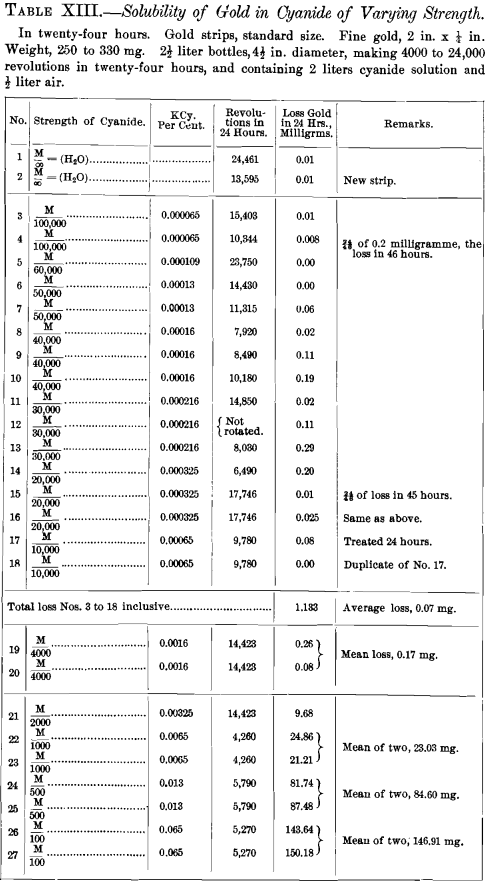
would at first lie in a layer of stronger solution that might have a slight solvent effect until the dilution was effected by rotation. The fact that the mean loss up to M/10,000 is only 0.07 mg.; that even at M/10,000 (Exp’t No. 18), no loss, and in No. 20 a loss of only 0.08 mg. was obtained, renders it extremely probable that the solution-loss up to M/10,000 or 0.00065 per cent is absolutely nil. Thus much is certain : these experiments demonstrate that for all practical purposes the cyanide of potassium, solution ceases to act at a strength below 0.001 per cent.
Going to higher strengths we find a sudden jump at M/2000 ; here the loss has risen to 9.68 mg., and beyond this it rapidly increases; the strips in the M/100 or 0.065 per cent, solution being eaten through in 24 hours.
The next set of experiments was devised to show the effect of a smaller volume of cyanide solution, and an unlimited supply of air. The same bottles as before were used, but they contained only 500 cc. of solution, and were left open to the air so that the latter was free to enter. The results, as shown in Table XIV., are in general the same as before. No appreciable loss occurs up to M/2000, but at that point, and for greater strengths, the loss rapidly increases, finally rising a little higher than before. In Experiments Nos. 4 and 6 the entire solution was filtered and the washed filter was scorified and cupelled. In No. 4, where the gold-loss was 0.07, none was found. In No. 6 (the loss being 0.23 mg.), 0.02 mg. of abraded gold was found. Whether the rest was fine enough to pass the filter, or was dissolved before the strong solution was diluted, is a conjecture.
The next experiments were made without agitation and in the following manner: The gold strips were suspended in perforated glass tubes just below the surface of the solution, so that although the solution was at rest, circulation by convection was possible. The volume of the solution was 250 cc. The time of action was in each case 48 hours. The losses are
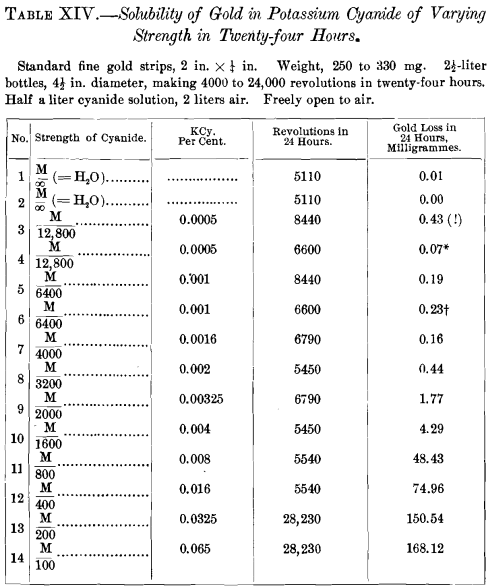
given in Table XV.; they are somewhat smaller than before, and again negligible below M/1000. In this case M/2000 was not determined. The advantage of the position near the surface near the air is well shown in comparing Nos. 5 and 8. When the gold was suspended near the surface of a M/400 solution, the loss was 21. 44 mg. in 48 hours, or nearly half a milligramme per hour; when the strip was put at the bottom of the same volume of a similar solution, the total loss in the same time was only 8.70 mg., or hardly one-third as much.
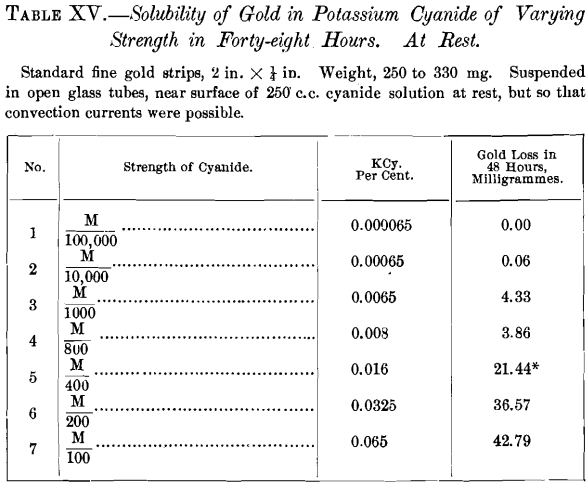
All these results have been plotted together with the voltage of the gold in curves a, b, c and d, in Fig. 20. According to the voltage-curve the voltage becomes zero for a cyanide solution of 0.00675 M, or about 0.044 per cent., and it is a curious fact that this is very near the limit of strength that practice has so far justified. Nevertheless, solutions as low as 0.01 per cent., and even less, have been employed in practice; and my experiments show that the solution acts perceptibly down as low as M/2000 or 0.00325 per cent., and perhaps to M/4000 or 0.0016 per cent. KCy.
An Apparent Contradiction
Although it will be seen that the solubility-curves all follow very closely the voltage-curve, the fact that action does not cease for the zero of potential of gold in KCy solution seems to contradict the Nernst law. But this contradiction is only apparent and not real. It is part of the new theory that (except the infinitesimal amounts necessary to produce the state of static tension) ions cannot come into ex-
istence or disappear except in pairs. That is, for every ion with a positive charge of electricity there must be one with an equal negative charge. Hence, when a positive ion appears, another positive ion must disappear, or else a negative one must also appear simultaneously. (In the case of ions with varying valency, an ion having a double or treble valency is, of course, equivalent to two or three oppositely electrified univalent ions.)
For instance, in my experiments for determining the electromotive force of gold in potassium cyanide solution against the normal electrode, the couple is composed of
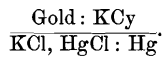
When the gold dissolves, the positive gold ions travel from the gold with their positive charge, and in order that the solution may continue, a similar flow of positive ions must continue by means of potassium ions through the solution to the mercury. The potassium ions finally drive out some of the mercury ions which precipitate into the mercury forming the electrode, at the same time giving up their charge of positive electricity to it. Simultaneously there is a corresponding flow of negative ions in the opposite direction. Thus: first chlorine and then cyanogen move in the opposite direction to meet the gold, and the latter forms with the cyanogen the complex negative ion (AuCy2) (—). Thus: Au (+) +Cy (—) + Cy (—) = (AuCy2) (—).
The action of this couple will go on so long as the electromotive force of the combination is greater than zero, and, as we have seen in my experiments, long after the electromotive force of the gold in the dilute cyanide solution has become zero. For the tendency of the mercury ions to discharge into the mercury electrode can only affect its purpose and cause a current by the simultaneous solution of the gold. That is, the tendency of the positively electrified ions of mercury to discharge themselves can cause the gold to dissolve long after its own electromotive force has ceased.
The Effect of Hydrogen Peroxide
Conclusions
Whatever may be the nature of the objections that may be raised against the final acceptance of the modern electrolytic theory in its present form, it will, I think, be conceded that the following conclusions may be fairly drawn from the foregoing:
- That the new electrolytic theory explains in a remarkably complete manner the reason for the departure of metals immersed in cyanide solutions from the sequence of electromotive force which they present in acid solutions.
- That it is the only theory ever presented that gives any clue to the remarkable aberration of cyanide solutions from all the usual chemical analogies.
- That it explains in an entirely adequate manner the reason for the reactions that go on when gold, silver and other metals are dissolved and precipitated from cyanide solutions.
- That the determination of the electromotive force of the metals in cyanide solutions under different conditions offers a means of research that is likely to be of great practical utility in determining the direction and intensity of chemical reactions, under fixed conditions, or in following them under changing conditions, just as they occur.
- That the differences of electromotive force of metals in dilute cyanide solutions do not give much support to the so-called “ selective affinity of dilute cyanide solutions for gold,” the only common metal that shows any indication of such favorable action being copper.
- It is probable that, in the absence of external electromotive forces, an aerated cyanide solution less than M/10,000 or 0.00065 per cent, is without action on metallic gold.
- That for all practical purposes, an aerated cyanide solution of less than 0.001 per cent, is without action on metallic gold.
This study has led apparently far afield from the practical side of the cyanide process; yet I hope that it may be of service in at least calling attention to the work of others who have toiled for many years in attempting to clear up some of the most subtle questions that have ever taxed the human mind. For I am firmly convinced that, in the long run, such work is always of the greatest practical service. In these days, the words of Ostwald have certainly come true:
“ The science of today is the practice of tomorrow.”
